

Using the integration of uv formula ∫u.dv = uv- ∫v du we get ∫ u (dv/dx) (dx) = ∫ d/dx (uv) dx – ∫ v (du/dx) dxĪs a result, the Integration of the UV formula may be obtained. Perform the integration on both sides with regard to x, Let’s take a close look at the following example of applying the chain rule. Higher Maths Resources Essential Skills 22, Further Differentiation Essential Skills 23, Further Integration Grade Booster Paper 4, Stationary Points Grade. This skill is to be used to integrate composite functions such as. This is known as the reverse chain rule since it is found by reversing the chain rule by integration. Exponential and logarithmic functions are used to model population growth, cell growth, and financial growth, as well as depreciation, radioactive decay, and resource consumption, to name only a few applications. When we use the product rule of differentiation, we will obtain the following results:Īfter some reorganization of the phrases, we have, By recalling the chain rule, the Integration Reverse Chain Rule comes from the usual chain rule of differentiation. 5.6: Integrals Involving Exponential and Logarithmic Functions. We have two functions, u and v, and that y is the solution to the equation uv. Using the product rule of differentiation, we will construct the formula for the Integration of UV. However, this type of Integration is also valuable on its own.į the two functions are of the type u,v then the formula for the Integration of u and v may be written as follows: Integration by parts is a specialised technique of Integration that is beneficial in various situations, including when two functions are multiplied together.

Let’s look at how the UV formula is integrated and what it may be used for. There are two forms of this formula: uv dx u v dx - (u' v dx) dx (or) u dv uv.
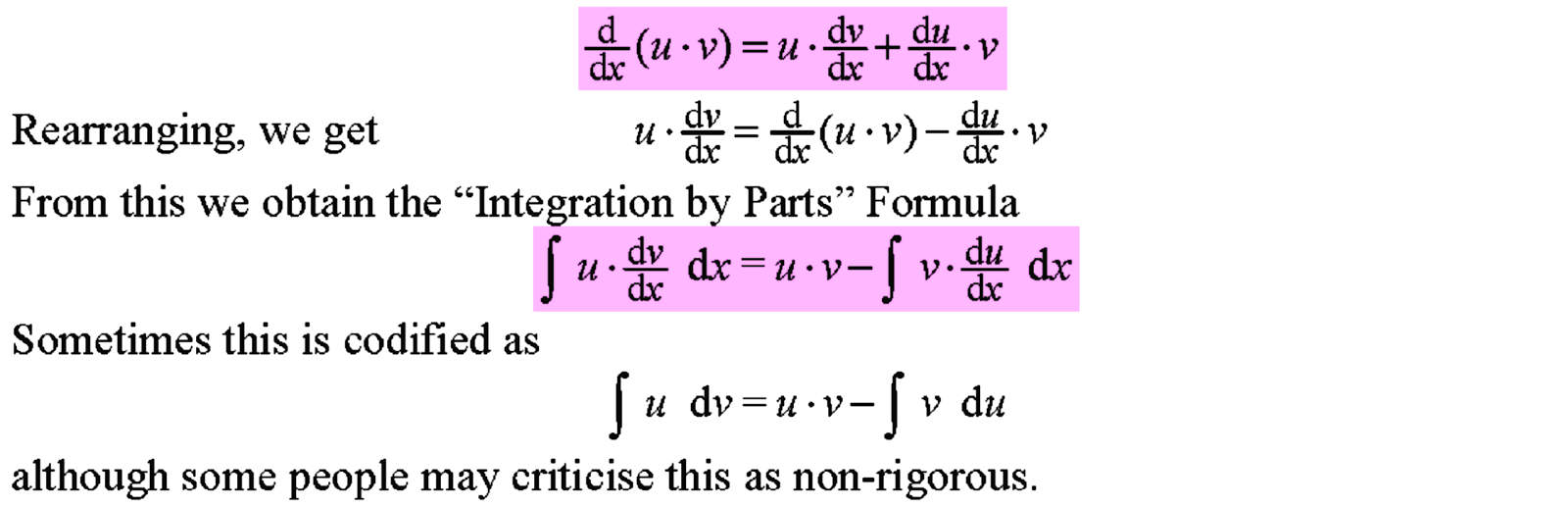
Integration of uv formula is a convenient means of finding the integration of the product of the two functions u and v. Hence, the term “integration by parts” or “the product rule of integration” refers to the process of integrating a UV formula. Expert Maths Tutoring in the UK - Boost Your Scores with Cuemath. We can use this method to find an integral value when it is set up in the special form. To express the provided integral, we use the differential of a product of functions to extend it. In calculus, the integration by substitution method is also known as the Reverse Chain Rule or U-Substitution Method. This formula for integrating UV may be used for algebraic equations, trigonometric ratios, and logarithmic quantities. So in essence your argument is on the right track. Using the notation of indefinite integrals we have that: f ( g ( x)) g ( x) d x F ( g ( x)) f ( u) d u u g ( x).
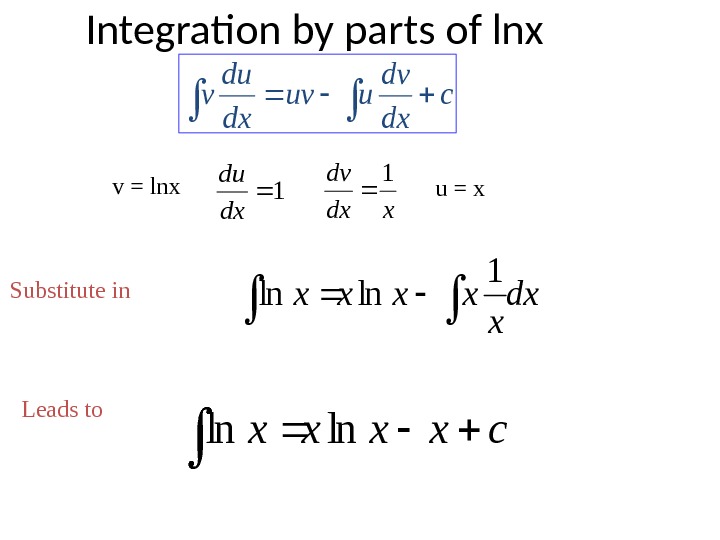
Thus h is an antiderivative for f ( g ( x)) g ( x). It is the counterpart to the chain rule for differentiation, and can loosely be thought of as using the chain rule 'backwards'. Hence, the term integration by parts or the product rule of. In calculus, integration by substitution, also known as u-substitution, reverse chain rule or change of variables, is a method for evaluating integrals and antiderivatives. Using the UV formula to obtain the product of the two functions u and v is a straightforward way to discover the Integration. Using the chain rule we get that: h ( x) F ( g ( x)) g ( x) f ( g ( x)) g ( x). To express the provided integral, we use the differential of a product of functions to extend it.


 0 kommentar(er)
0 kommentar(er)
